Boxing the Blocks

There's nothing wrong with your nephew. There is no way to pack the twenty-seven blocks into the box.

This fact was proven by the mathematician de Bruijn who demonstrated that collection of bricks with the dimensions a x ab x abc can only be efficiently packed into a volume measuring ax x aby x abcz. If you can visualize the relationship between these volumes, you can see that in that case, the bricks can be packed uniformly- all with the same orientation. So if a brick with dimensions a x ab x abc can be packed into a volume, it can be packed simply.

Now in the case of your Nephew's blocks, can this be simply demonstrated without knowing of de Bruijn's proof?

Consider a block packed with red and blue 2 x 2 x 2 bricks, as shown below:

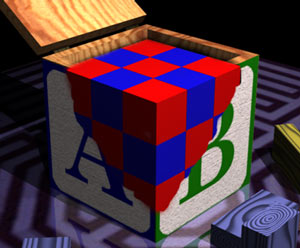
If you were to place your nephew's blocks in the box, each block would occupy four cubic inches of "blue brick space" and four cubic inches of "red brick space." But there are more red bricks than blue bricks. So no matter how the 4 x 2 x 1 blocks are placed in the box, one will always be left that can't fit in the remaining space.